The Pythagorean comma
The Pythagorean comma demonstrates that our tonal system is not perfectly consistent but has a gap whose form and cause I will describe in this post. The comma is relevant in terms of both ourpythagorean comma musical practice, since it has very specific effects, and of philosophy and science, since it is typical of the problems that we observe in the interplay of our three worlds (according to Penrose). Thus it is a topic that is not solely relevant to musicians but also to people who are interested in the question as to how mathematics (ideal world), physics (physical world) and our experience (mental world) relate to each other.
To begin with, I’ll explain why this comma occurs.
Adding intervals
What happens if we add two intervals, for instance a fifth and a fourth? We will see that such an addition works perfectly in some cases but leads to problems in others. This is where the origin of the comma lies and the reason which led to the tempered mood. Why do problems occur here at all? This is what I would like to have a closer look at now, later also at the solution to the problem.
For intervals, adding means multiplying
Can we add intervals just like that? The problem is that we sense the intervals as behaving linearly but the frequencies do not increase linearly, but exponentially.
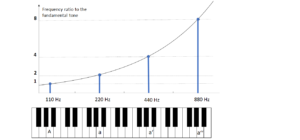
Between tone A (110Hz) and tone a (220 Hz), there is a distance of 110 Hz. This distance corresponds to an octave – but only there! If we now measure the distance from tone a to tone a’, which again corresponds to an octave, we do not get 110Hz, but 200Hz. And from a’ to a”, we already get 880Hz. This is because the frequencies do not increase in a linear fashion, but exponentially, as depicted in Fig. 1.
This exponential behaviour is where the root of the problem lies and which has led European musicians to try out various tempered moods, with the equal temperament ultimately prevailing. The exponential behaviour has the following unexpected effect: When we add intervals, we cannot add, but have to multiply their frequency ratios, and when we subtract them, we have to divide them. The operations shift from addition/subtraction to multiplication/division.
This shift of operations is known in other domains, too: Before pocket calculators and computers, technicians used so-called logarithmic tables and slide rules, which are based on precisely this shift. This effect can also be observed in the combinatorial explosion and in the progress of epidemics.
Examples of interval additions
What always interests us when we look at intervals is the ratio of the frequencies of the two tones of the interval, i.e. the fraction between the frequency of the higher tone divided by the frequency of the lower tone: X = f2/f1. This fraction determines the intervals which we perceive.
Octave and octave
Cf. Fig. 1: we add two octaves, for example A-a and a-a’.
The first octave (A-a) measures 220/110 = 2.
The second octave (a-a’) measures 440/220 = 2.
The two octaves together make 2×2 = 4.
The multiplication is correct here since a’ is 4 times as fast as A (440Hz / 110Hz). Thus two octaves result in a quadruplication of the basic frequency.
Fifth and fourth
The fifth corresponds to a frequency acceleration to 3/2,
the fourth to an acceleration to 4/3.
The fifth and the fourth together result in 3/2 * 4/3 = 12/6 = 2.
2 corresponds to an octave. Thus mathematically, a fifth and a fourth together result in precisely one octave. Here, too, the mathematics is in line with what we expect to hear.
Further additions to an octave
Minor third plus major sixth = 6/5 * 5/3 = 30 /15 = 2.
Major third plus minor sixth = 5/4 * 8/5 = 40 /20 = 2.
Above an octave
Two fifths: 3/2 * 3/2 = 9/4 = 2.25.
2.25 is bigger than 2, i.e. we have left the range of an octave. Where have we ended up? With a ninth. A ninth is an octave plus a major second. Will this work out? We calculate:
Octave plus major second = 2 * 9/8 = 18/8 = 9/4 = 2.25.
Yes, it works! When we add two intervals, we must multiply their fractions.
Subtraction becomes division
Of course, it also works the other way round: we can subtract one interval from another. Then, we have to divide:
An octave minus a fifth = 2 : 3/2 = 4/3 = a fourth.
A fifth minus a fourth = 3/2 : 4/3 = 9/8 = a major second.
Why do these calculations work out?
I was surprised to see that despite the shift in arithmetic operations, the calculation provides precisely the results which a musician expects. How can this be? After all, a multiplication is something completely different from an addition. Why can we calculate like this all the same?
The fact that the calculations work out so perfectly is not a matter of course, and they do not always work out perfectly, either, as we are about to see. In the above examples, though, they work out, and this is only the case because we made a clever choice of intervals. The reason for this is that in the course of many millennia, countless musical people developed a tonal system which allows for precisely this.
As represented in earlier posts, the choice was everything but random, but made according to the criteria for resonant musical scales. And we noticed that intervals are resonant when they represent fractions with integral numerators and denominators and that it is necessary for a simultaneous resonance of several intervals that both numbers are as low as possible and, in particular, do not contain a prime higher than 5 in the prime factorisation.
These restrictive conditions enable us to reduce the fractions when we compare the intervals with each other. High numbers, and high primes in particular, do not lend themselves to reduction. As we have seen in the above examples, however, reduction is a great help for us.
This is most important for scales and chords which both have more than just two tones. Let us look at a combination of two intervals into a bigger interval. If we calculate the combined interval, we have to multiply numerators and denominators, which will soon result in very high numbers. High numerators and denominators, however, mean low resonance. If we are now able to reduce the resulting fraction, the reduced numbers of numerator and denominator will show that resonance still is going to take place. Therefore combinations of several intervals – a property of scales and chords – may lead to new intervals that still are resonant – if it is possible to reduce. And only in this way will a combination of two resonant intervals result in another resonant interval. And only then will we remain within our resonant musical scale.
Therefore, reducing is quite practical, especially if we combine several intervals.
– Only, it does not always work out.
It would be too nice…
The shift from linear to exponential growth leads to problems faster than we expect. Even the simplest calculations do not work out:
Major second plus minor seventh = 9/8 * 9/5 = 81 / 40 = 2.025.
We do not actually expect 2.025, but 2, i.e. a pure octave.
Major third minus major second = 5/4 : 9/8 = 40/36 = 10/9 = 1.111.
We actually expect a major second, i.e. 9/8 = 1.125.
A fourth and a fourth = 4/3 * 4/3 = 16/9 = 1.777.
We actually expect a minor seventh = 9/5 = 1.800.
As you can see, our expectation that calculations with intervals work out has been disappointed. Only very few interval combinations allow for “pure” calculations, and then only because we have chosen our scale system so well. All the other interval combinations do not work out. Usually, the discrepancies are not very big, but they still exist distinctly. The phenomenon that interval combinations do not work out has become famous under the name of Pythagorean comma.
The Pythagorean comma
Pythagoras already knew that natural, i.e. well-sounding intervals can be traced back to simple fractions with low numbers. As we know, the simplest intervals with the lowest figures are the octave (2/1), the fifth (3/2) and the fourth (4/3).
As we have seen above, two fifths together result in a ninth. How many fifths does it take to return to the fundamental tone? Let’s look at this from the C and examine how many fifths it takes to reach another C:
C – G: first fifth
G – D: second fifth
The whole sequence is as follows:
C – G – D – A – E – H – F# – C# – Ab – Eb – B – F – C
We thus have twelve fifths. The low and the high Cs are seven octaves apart. Thus the upper C, calculated through the fifths is 3/212 = 129.746 and calculated through the octaves is 27 = 128. The discrepancy between the two calculations is 129.746 : 128 = 1.0136.
This small discrepancy of 0.0136 is the Pythagorean comma.
Placing the comma into a larger context
The Pythagorean comma is inevitable and ultimately derives from the fact that mathematically speaking, we are sailing under two different flags here, namely one which adds and another which multiplies. Our thinking, which takes its primary orientation from matter and space, is accustomed to the linear calculations with which lengths are measured. We also look at the intervals in this manner. However, these work through frequencies and their mutual ratios, and those are not linear, but exponential.
Incidentally, this is not the only field where our habit of thinking in a linear way becomes a trap. Many processes progress exponentially; examples are the combinatorial explosion, the observation of a collective of several objects, and the progress of an epidemic, social trends, etc. As soon as what happens becomes complex, exponential conditions should not surprise us.
This leads us back to the fundamental issue of this series, namely the relations between the three worlds. What is the role of mathematics for physics and our minds? I will leave this question unanswered here and remain in the field of music for the time being. In the next post, I will explain the advantages provided by our solution to the comma problem, namely the equal temperament.
Conclusion
Calculations with intervals are made by multiplying and dividing the fractions of their frequencies.
- This is in contradiction to our intuitive idea that this involves additions and subtractions.
- For this reason, most “additions” and “subtractions” of two intervals do not result in the pure intervals we expect.
- Only very few additions/subtractions of intervals result in other pure intervals. This is only possible if the fractions of the intervals involved can be reduced.
- This is subject to the same rules as those applying to the determination of resonant scale tones: numerators and denominators must be low numbers; higher primes, in particular, hamper resonance and calculability.
- The Pythagorean comma is an expression of this fundamental mathematical incompatibility of linearity (addition) and exponentiality (multiplication).
- Thus the Pythagorean comma places a limitation on the pure intonation.
We will soon see how the problem of the Pythagorean comma is solved by means of the equal temperament. But before that, we will have a look at the distribution of the tones within the octave in just intonation.
This is a post about the theory of the three worlds.